The complicated: I didn't get much farther. Not so much because I didn't understand it, but The complicated: I didn't get much farther.
The complicated: My arithmetical skills blow on the same level as Johann Kepler's.* If I don't rewrite the determinant as
I will blow the sign and the whole problem. Which is probably where my issues were in 10th grade. So I'm pausing to practice, catch up the blog (for real this time, I have a new toy perfectly capable of the job much quicker than computer Photoshopping). But hey, I made it this far, didn't I? That's a pile of awesomeness. I can look at a matrix and say, "What do you want me to do with that?" I don't think synthetic division relates to creative accounting. And I remember the almighty quadratic equation:
I will blow the sign and the whole problem. Which is probably where my issues were in 10th grade. So I'm pausing to practice, catch up the blog (for real this time, I have a new toy perfectly capable of the job much quicker than computer Photoshopping). But hey, I made it this far, didn't I? That's a pile of awesomeness. I can look at a matrix and say, "What do you want me to do with that?" I don't think synthetic division relates to creative accounting. And I remember the almighty quadratic equation:
It's just going to take a little longer than I thought.
*Johann was struggling to explain a mystery of the planets using the "five perfect shapes" of Pythagorean geometry. Perfect in the same way ancient Greeks considered octaves, fifths, and fourths perfect, but have any on your theory project and you'll regret it. He struggled to find an arrangement that made sense. He actually found the correct answer...and screwed up the arithmetic. It's okay, Kepler. I know how you feel.

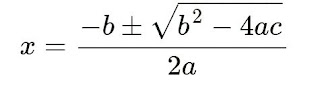
No comments:
Post a Comment